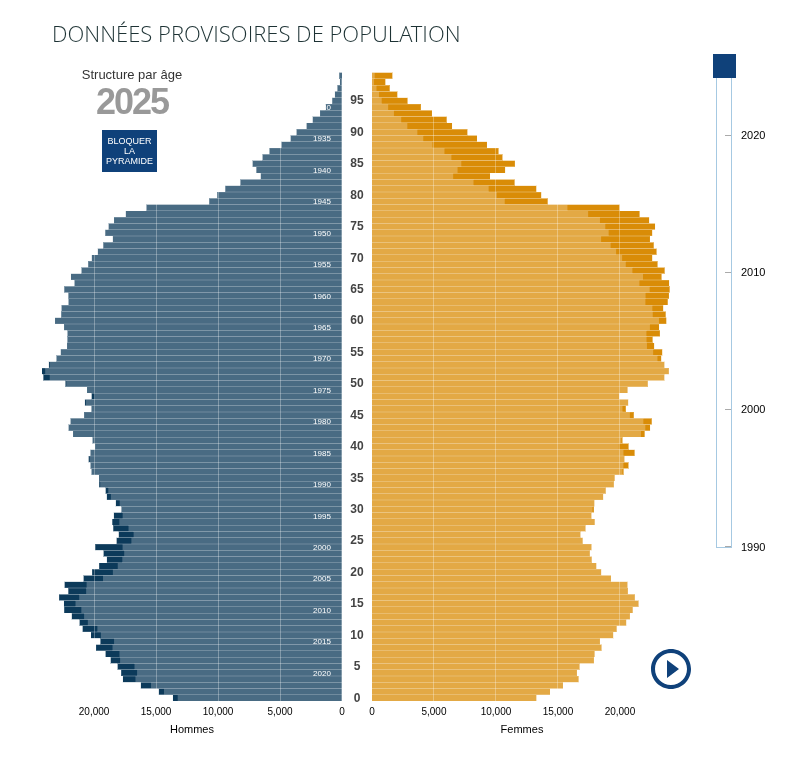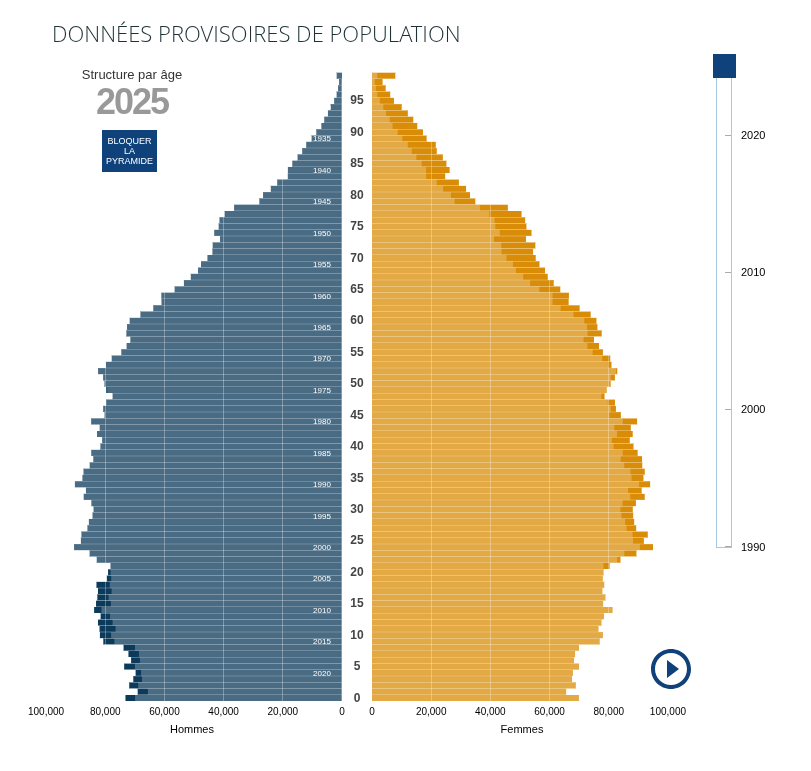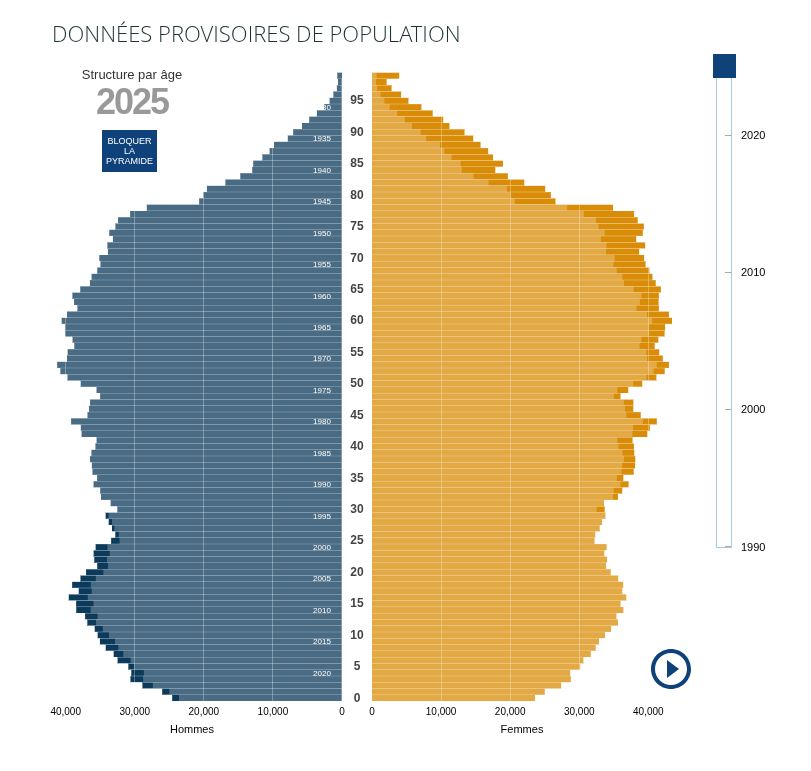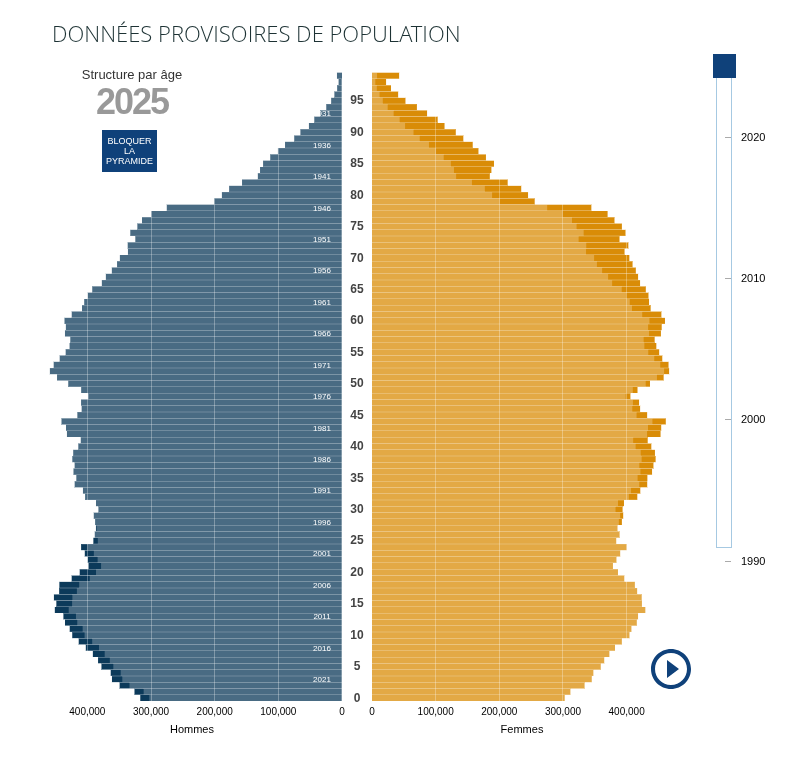
Il est intéressant de jeter un oeil aux données démographiques et notamment à la pyramide des âges publiée par l'Institut national de la statistique et des études économiques (INSEE), analysée par l'INSEE mais aussi par l'Institut national d’études démographiques (Ined).
Depuis 2010, la baisse de la natalité est marquée. Le système éducatif a déjà commencé à se vider progressivement de ses élèves. La vague touchera l'université juste avant 2030, et cela va coïncider de peu avec des départs massifs à la retraite. De quoi bien occuper gouvernants et gouvernés. Ces dynamiques globales n'empêchent pas des disparités régionales et locales.