Djalil CHAFAÏ (شافعي جليل)
Mathematician - University professor
- Université Paris-Dauphine - PSL • CEREMADE • Place du Maréchal de Lattre de Tassigny, 75775 Paris Cedex 16
- Made available (« mis à disposition ») by Dauphine to École normale, from September 2021 to August 2031
- Email. firstname@lastname.net (without diacritics)
Current duties include...
- Directeur scientifique du RNBM (2021 - 2025) après Frédéric Hélein
Research topics up to now
- Geometric and probabilistic functional analysis, concentration, convexity, isoperimetry
- Random matrices, random graphs, free probability, and high dimensional phenomena
- Convexity and positivity, variational analysis, potential theory
- Asymptotic analysis, exact solvability, special functions
- Stochastic models and stochastic inverse problems:
- Mathematical Biology (pharmacology, biostatistics)
- Mathematical Physics (spin systems, interacting particle systems, statistical physics)
- Data Science (data streams processing, queuing, machine learning, decision making)
- Earth Science (variational assimilation in meteorology)
- Random structures and algorithms, stochastic simulation
- Markov processes/semigroups, their equilibrium/geometry
- Sobolev type inequalities and partial differential equations
- Poisson and Gauss measures, heat kernels, limit theorems
- Boltzmann-Shannon and Voiculescu entropies, information theory, operator theory
Pictures
Some pictures illustrating my research, created mostly with Octave, Python, or Julia. Enjoy visual mathematics!
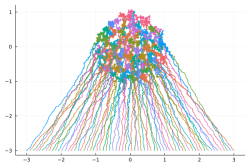
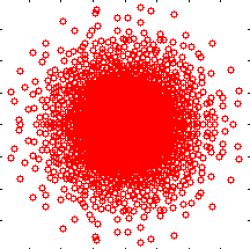
- Left: Spectrum of a high dimensional square matrix with heavy tailed (infinite variance) iid entries:
Right: Voronoi cells of the spectrum of a high dimensional matrix with iid Gaussian entries:
More in arXiv:1006.1713 and in arXiv:1109.3343 - Trajectories of an eight particles Hamilton-Monte-Carlo algorithm for a one-dimensional log-gas:
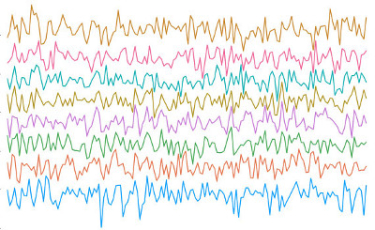
More in arXiv:1806.05985 - Phase portrait of the reciprocal polynomial of the characteristic polynomial of a high dimensional Gaussian matrix (left: complex, right: real):
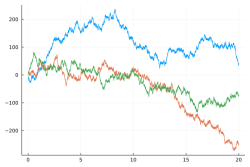
More in arXiv:2012.05602 and Blogpost - Left: three independent real Brownian motion (time in horizontal axis):
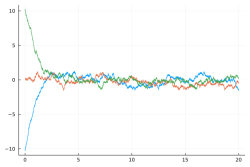
Center: the same BMs with a quadratic confinement making them three independent Ornstein-Uhlenbeck (OU) processes:
Right: the same OUs with singular repulsion making them a single three particles Dyson-Ornstein-Uhlenbeck process:
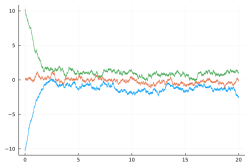
More in arXiv:2107.14452 Talk slides - Trajectory of a planar Dyson Ornstein-Uhlenbeck process with 66 particles started from a uniform grid on circle or line:
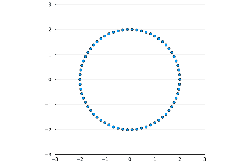
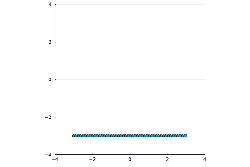
Click on picture to see animation. More in arXiv:1706.08776 and in Blogpost - Equilibrium measures of planar Coulomb gas with various conditionings on linear statistics:
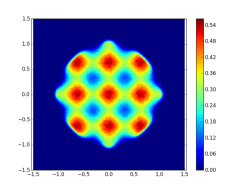
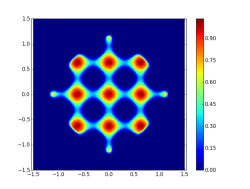
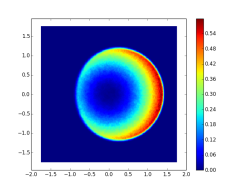
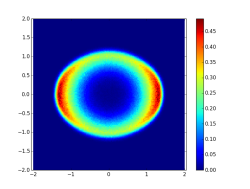
These pictures are courtesy of my co-author Grégoire Ferré. More in arXiv:1907.05803 - Equilibrium measure in dimension $4$ for $\log\left\Vert\cdot\right\Vert_2$ repulsion and $\left\Vert\cdot\right\Vert_4^4$ confinement, projected on first three coordinates:
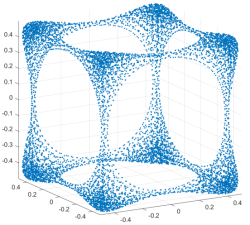
This picture is courtesy of my co-author Robert S. Womersley. More in arXiv:2206.04956
Doctoral and postdoctoral advisory
- Current doctoral or pre-doctoral students
- Samuel Chan-ashing (2024-, Paris-Dauphine - PSL, with Quentin Cormier)
Long time behavior of diffusion processes : cutoff phenomenon and metastability - Rémi Bonnin (2024-, Aix-Marseille Université, with Charles Bordenave)
High dimensional random tensors - Kewei Pan (2023-, Toulouse, with Guillaume Cébron)
Around Boolean entropy, random matrices, and Coulomb gases - Quentin François (2022-, Paris-Dauphine - PSL, with Pierre Tarrago)
Random Matrices : high dimension and exact solvability
- Former postdoctoral researchers
- Guillaume Dubach (2022-2023, FSMP)
Probability theory, especially random matrices
Actuellement professeur Monge à l'École polytechnique
-
- Hugo Magaldi (Paris-Dauphine - PSL 2022, with Laure Dumaz)
Étude des beta-ensembles et leur limite à haute température
Actuellement ingénieur - Jeanne Boursier (Paris-Dauphine - PSL 2022, with Sylvia Serfaty)
Quelques problèmes de mécanique statistique pour les gaz de Coulomb et de Riesz
Postdoctoral fellow, J.F. Ritt Assistant Professor, Columbia University, New York - Josué Corujo (Paris-Dauphine - PSL 2021, with Simona Grusea)
Multi-allelic Moran models and quasi-stationary distributions
Actuellement maître de conférences à l'Université Paris-Est Créteil - Ezéchiel Kahn (École des Ponts ParisTech 2021, with Benjamin Jourdain)
Matrices de covariance : diffusions, probabilités libres, et apprentissage profond
Actuellement haut fonctionnaire du Corps des Ponts, des Eaux, et des Forêts - David García-Zelada (Paris-Dauphine - PSL 2019)
Aspects géométriques et probabilistes des gaz de Coulomb
Actuellement maître de conférences à Sorbonne Université - Raphaël Butez (Paris-Dauphine - PSL 2017)
Polynômes aléatoires, gaz de Coulomb, et matrices aléatoires
Actuellement maître de conférences à l'université de Lille - Marie-Noémie Thai (Marne-la-Vallée / Paris-Est 2015, with Amine Asselah)
Processus de Fleming-Viot, distributions quasi-stationnaires et marches aléatoires en interaction de type champ moyen
Actuellement enseignante à l'École supérieure d'ingénieurs Léonard-de-Vinci - Bertrand Cloez (Marne-la-Vallée / Paris-Est 2013)
Comportement asymptotique de processus avec sauts et applications pour des modèles avec branchement
Actuellement chargé de recherches à l'INRAE - Julie Champion (Toulouse 2013, with Franck Barthe)
Sur les algorithmes de projection en entropie relative avec contraintes marginales
Actuellement professeure en classes préparatoires aux grandes écoles - Julie Antic (Toulouse 2009, with Didier Concordet)
Nonparametric methods for population PK and/or PD
Actuellement ingénieure dans l'industrie spatiale
Agenda
Scientific life in Dauphine and École normale
Conferences, schools, days, ...
Underlined item if participation to the organization or scientific committee. Standard seminars/colloquiums are not listed.
- 2024-09. Robust Optimization and Simulation of Complex Stochastic Systems (ICERM Providence)
- 2023-11. Quarante ans du RNBM (Luminy)
- 2022-12. Conference on universality (Paris)
- [Archive] 2020-05. Random Matrices and their Applications (New York) < Covid-19 - Onlined
- [Archive] 2018-05. Random Matrices and their Applications (Kyoto)
- [Archive] 2018-05. Concentration of measure and its applications (Cargèse)
- [Archive] 2017-12. Journée algorithmes stochastiques à Paris-Dauphine (Paris-Dauphine)
- [Archive] 2015-06. Journée CEREMADE-LAMSADE-LEDa 2015 (Paris-Dauphine)
- [Archive] 2015-01. Workshop on random matrices and their applications (Hong Kong)
- [Archive] 2014-12. États de la recherche en matrices aléatoires (IHP Paris)
- [Archive] 2014-08. Journées MAS 2014 (Toulouse)
- [Archive] 2014-03. Recent advances on log-gases (IHP Paris)
- [Archive] 2014-03. Journée Hawkes à Paris-Dauphine (Paris-Dauphine)
- [Archive] 2013-05. School on threshold phenomena and random graphs (IHP Paris
- [Archive] 2012-10. Workshop on random matrices and their applications (Telecom Paris)
- [Archive] 2012-03. Journées Processus de Markov Déterministes par Morceaux (Marne-la-Vallée)
- [Archive] 2010-05. Workshop on Probability and Geometry in High Dimensions (Marne-la-Vallée)
- [Archive] 2008-06. Journées Statistiques du Sud 2008 (Toulouse)
Projects membership (only running projects)
- Réseau Thématique “Mathématiques et Physique” Axe Matrices Et Graphes Aléatoires (MEGA) funded by CNRS
Papers
You may read Publications: science, money, and human comedy on my blog.
Digital footprint in professional data bases :
Partial list of publications automatically provided by arXiv :
[Loading myarticles...]
Books
Authored books
- Topics in statistical mechanics
With Ewain Gwynne, Nina Holden, Xin Sun, Arnaud Le Ny, Sébastien Ott, Yvan Velenik, Rémi Rhodes, Vincent Vargas
Edited by Cédric Boutillier, Béatrice de Tilière, Kilian Raschel
Panoramas et Synthèses 59, Société Mathématique de France (SMF), (2023) 229p.
Chapter on arXiv
- Modern Aspects of Random Matrix Theory
With Charles Bordenave; Alice Guionnet; Alan Edelman, Brian Sutton, Yuyang Wang; Mark Rudelson; Terence Tao, Van Vu (Editor)
Proceedings of Symposia in Applied Mathematics (PSAPM) 72 American Mathematical Society (AMS), (2014) 172p.
- Interactions between compressed sensing, random matrices, and high dimensional geometry
With Olivier Guédon, Guillaume Lecué, and Alain Pajor
Panoramas et Synthèses 37, Société Mathématique de France (SMF), (2012) 182p.
Draft (PDF) · Errata (PDF)
- Sur les inégalités de Sobolev logarithmiques (in French)
With Cécile Ané, Sébastien Blachère, Pierre Fougères, Ivan Gentil, Florent Malrieu, Cyril Roberto and Grégory Scheffer
With a preface by Dominique Bakry and Michel Ledoux
Panoramas et Synthèses 10, Société Mathématique de France (SMF), (2000) xvi+217p.
Photo of S.O. Bologs · Private access for sobologs
Preface · Errata (PDF) · Expanded first chapter on HAL
Edited books
- Advanced Topics in Random Matrices
Proceedings of the États de la recherche 2014, Société Mathématique de France, held in Institut Henri Poincaré, Paris.
By Florent Benaych-Georges, Charles Bordenave, Mireille Capitaine, Catherine Donati-Martin, Antti Knowles
Edited by Florent Benaych-Georges Djalil Chafaï, Sandrine Péché, and Béatrice de Tilière
Panoramas et Synthèses 53, Société Mathématique de France (SMF), (2018) xii+204p.
- Covid-19 | Regards croisés sur la crise
Recueil de textes pluridisciplinaires rassemblés par Bruno Bouchard et Djalil Chafaï
Éditions électroniques de l'Université Paris-Dauphine - PSL, 113p (2021)
Authored pedagogical books
- Aléatoire (dont une introduction aux matrices aléatoires)
En collaboration avec Sylvie Méléard et Christophe Giraud
Actes des journées X-UPS 2013
Édités par Pascale Harinck, Alain Plagne, et Claude Sabbah
Éditions de l'École Polytechnique (2013) 122p.
Livre (papier ou PDF) · Exposé (PDF) · Questions/réponses (Blog)
- Modélisation stochastique et simulation (cours et applications)
En collaboration avec Bernard Bercu
Mathématiques appliquées pour le Master - Collection Sciences Sup
Société de Mathématiques Appliquées et Industrielles (SMAI)
Éditions Dunod (2007) 352p.
Errata (PDF) · Codes (ZIP)
- Recueil de modèles aléatoires
En collaboration avec Florent Malrieu
Collection Mathématiques & Applications Volume 78 Société de Mathématiques Appliquées et Industrielles (SMAI)
Éditions Springer (2016) XIII+398p (Mathematical Reviews)
ISBN 978-3-662-49767-8 (Print) 978-3-662-49768-5 (Online)
Version papier et électronique chez Springer et version électronique sur libgen
Rappels de cours (43p) + errata version Springer + codes (Julia, Octave, Python, Scilab)
Version électronique auteurs post-publication sur HAL
- Probabilités - Préparation à l'agrégation interne
En collaboration avec Pierre-André Zitt
Auto-édition, version électronique gratuit et version papier vendue à prix coûtant (163p.)
Version électronique en couleur disponible gratuitement sur HAL, éditions 2016, 2017, 2020
Version papier noir & blanc vendue à prix modique et coûtant (<5€) sur Amazon France, éditions 2016, 2020 :
Documents
Talk slides and videos
- Autour de la théorie de l'information
Exposé pour un après-midi mathématique à destination des lycéens, École normale supérieure, Paris, 2024) : PDF - Cutoff for Dyson Ornstein Uhlenbeck process with a focus on distances in high dimension
Talk slides (Focus Days @ LPENS, Paris, 2024) : PDF - Mini-course on logarithmic Sobolev inequalities
Lecture notes (MEGA@IHP and DMA@ÉNS, Paris, April and December 2024) : PDF - Riesz Energy Problems and Integral Identities - Unexpected phenomena for equilibrium measures
Talk slides (Paris, 2022) : PDF - Probabilités, analyse, et physique statistique
Talk slides (ÉNS, 2021) : PDF - About random matrices
Talk slides (Stanford, 2021) : PDF - Aspects of Coulomb gases
Talk slides (Oberwolfach, 2019) : PDF - Dynamics of a planar Coulomb gas
Video capture (Providence, 2018) : Stream - Matrices aléatoires
Video capture (Orsay, 2016) : Stream - Au bord de certains systèmes de particules en interaction issus ou inspirés par des modèles de matrices aléatoires
Video capture (Collège de France, 2016) : Stream - About non-Hermitian random matrices
Talk slides (Cargèse, 2014) : PDF - About confined particles with singular pair repulsion
Talk slides (Toulouse, 2014) : PDF - The circular law
Talk slides (San Diego, 2013) : PDF - Graphes et matrices aléatoires - Quelques aspects
Planches d'exposé MAP-432 (École Polytechnique, 2012) : PDF - Matrices aléatoires - Quelques aspects
Planches d'exposé (Marne-la-Vallée, 2011) : PDF - Autour du théorème limite central
Planches d'exposé (Marne-la-Vallée, 2011) : PDF
Classes préparatoires aux grandes écoles
- Processus des restaurants chinois et loi d'Ewens
Écrit avec Yan Doumerc et Florent Malrieu
Paru dans RMS ex Revue de Mathématiques Spéciales, vol. 123 n°3 (2013)
Article : PDF
Unpublished reports and expository notes
These documents are (un)published in the prestigious Journal of Unpublished Results. I have not tried viXra for the moment.
- About the spectral analysis of large random Markov kernels
Talk notes (2012) : PDF - Singular values of random matrices
Rough lecture notes (2009) : PDF - Dimension d'entropie, d'après Guionnet and Shlyakhtenko
Notes d'exposé (2008) : PDF - Questions/réponses simples de calcul stochastique
Notes pédagogiques (2008) : PDF - Random projections, marginals, and moments
Rough expository notes (2007) : PDF - Inégalités de Poincaré et Gross pour mesures de Bernoulli, Poisson, et Gauss
Notes d'exposition (2005) : HAL - Quelques mots sur l'entropie
Notes incomplètes (2002, 2005) : PDF - Sur certaines mesures produit conditionnées
Notes d'exposé (2005) : PDF - Covariance de modèles d'interface et marches aléatoires en environnement aléatoire
Notes d'exposé (2002) : PDF - Un petit peu de grandes déviations
Exposé au séminaire étudiant (1999) : PDF
C'est lors de cet exposé qu'a germé l'idée d'écrire un livre collectif sur les inégalités de Sobolev logarithmiques, en suivant l'exemple du mémoire collectif « Grandes déviations sans larmes » écrit par des doctorants du laboratoire quelques années plus tôt. Notre projet a pris forme en février 1999, par un exposé, le premier d'une liste de dix, au groupe de travail du laboratoire. Le premier jet de notre manuscrit collectif était dans nos valises lors de l'école d'été de Saint-Flour 1999 ! S'en est suivi un long travail et maintes péripéties… - Elementary proof of inequalities on paths space for Lévy processes
Short note (2002) : PDF - Covariance matrices with prescribed null entries
In collaboration with Didier Concordet
Rough technical report (2006) : PDF - A note on functional inequalities for some Lévy processes
In collaboration with Florent Malrieu
Short note (2002) : PDF
Extended by Ivan Gentil and Cyril Imbert in two published papers: (2008) HAL1 and (2009) HAL2 - Contributions à l'étude de modèles biologiques, d'inégalités fonctionnelles, et de matrices aléatoires
Mémoire d'habilitation à diriger des recherches (2008) : Thèse en ligne - Sur les inégalités de Sobolev logarithmiques en théorie de l'information et pour des systèmes de spins conservatifs en mécanique statistique
Mémoire de Doctorat (2002) : Thèse en ligne
Selected texts and tribunes
- Vice-présidence du numérique : 2017-2020
Billet de blog daté de décembre 2020 - Modélisation et analyse quantitative
Billet de blog daté de novembre 2020 - Modelling
Blog post dated May 2020 - Formation à distance
Billet de blog daté de mars 2020 - Paris-Dauphine : coronavirus et numérique
Billet de blog daté de mars 2020 - Franchise universitaire et droit de grève
Billet de blog daté de mars 2020 - Annals of mathematics: probability and statistics
Blog post dated November 2019 - How to give a bad talk
Blog post dated September 2018 - Lutte des classes et domination sociale
Billet de blog daté d'avril 2017 sur le monde universitaire - Réflexions sur les frais d’inscription en licence à l’université
Billet de blog daté d'octobre 2016 sur les frais d'inscriptions à l'Université - Dauphine : frais de scolarité
Billet de blog daté de janvier 2016 sur les frais de scolarité à Paris-Dauphine - Starting point and curiosity
Blog post dated June 2010 - Publications: science, money, and human comedy
Blog post dated January 2012 - Mathématiques de l'aléatoire et physique statistique
Contribution au livre de la recherche pour les cinquante ans de Paris-Dauphine (2018)
Version en ligne (billet de blog) - Mathématiques, probabilités, algorithmes
Texte d'introduction à un texte de Pierre Monmarché, lauréat du Prix du Monde de la recherche 2015.
Paru aux Éditions Le Pommier dans un recueil consacré au Prix.
Version en ligne (billet de blog) - Coût des publications : propositions concrètes
Paru dans Gazette des Mathématiciens 139 Société Mathématique de France (SMF) (2014) 82-86 PDF
Paru dans Matapli 103 (2014) 85-90 Société de Mathématiques Appliquées et Industrielles (SMAI) PDF
Version en ligne (billet de blog) - Coût des publications : un exemple instructif
Paru dans Gazette des mathématiciens 137 Société Mathématique de France (SMF) (2013) 119-122 PDF
Lire également EJP-ECP: twenty years of freeness (billet de blog)
Teaching
Ce que l'on conçoit bien s'énonce clairement et les mots pour le dire arrivent aisément.
Nicolas Boileau, L'art poétique (1674) Chant I.
J'ajouterais quant à moi que la réciproque n'est pas forcément juste. Méfiez vous des beaux parleurs !
ÉNS (Master 1 S1) : Processus stochastiques
- PDF 2024-2025 Notes de cours (~125p.)
- PDF 2024-2025 Partiel sans correction
- PDF 2024-2025 Partiel avec correction
ÉNS (Licence 3 S1) : Topologie et calcul différentiel
- PDF 2023-2024 Notes de cours (~120p.)
- PDF 2022-2023 Examen sans correction
- PDF 2022-2023 Examen avec correction
- PDF 2023-2024 Examen avec correction
ÉNS (Licence 3 S2) : Phénomènes de grande dimension
- PDF 2023-2024 Notes de cours (~90p.)
ÉNS (Master 1 S2) : High Dimensional Probability
For the year 2021-2022, this course presents selected aspects of high dimensional probability, dealing with the properties of high dimension random vectors, matrices, and tensors.
- Course topics (mainly based on M2 lecture notes)
- Gaussian measures and Ornstein-Uhlenbeck process
- Poincaré and logarithmic Sobolev inequalities
- Concentration of measure and transportation of measure
- Convexity and Bakry-Émery curvature-dimension criterion for Langevin processes
- Brenier and Caffarelli theorems on transportation of measure
- Gaussian type isoperimetry
- Discrete settings
- Selected bibliography:
ÉNS (Master 1 S1) : Free probability and random matrices (reading group)
For the year 2021-2022, we read the book Free probability and random matrices by Mingo and Speicher, Fields Institute Monographs 35 (2017).
Ce groupe de travail accessible ouvre au monde des probabilités libres et des matrices aléatoires. Il est basé sur le livre de Mingo et Speicher intitulé « Free probability and random matrices ». Prenant ses racines dans la théorie des algèbres d'opérateurs, les probabilités libres se sont entrelacées au fil du temps avec les partitions non croisées et la combinatoire, les matrices aléatoires et les phénomènes de grande dimension, les applications à l'apprentissage statistique et aux télécommunications sans fil, la théorie des représentations des groupes, les groupes quantiques, le problème du sous-espace invariant, les principes de grandes déviations, le problème des sous-facteurs, et au-delà. Ce groupe de travail met un accent particulier sur la relation entre probabilités libres et matrices aléatoires, mais aborde également, potentiellement, les aspects algébriques d'opérateurs, combinatoires, et analytiques de la théorie. Ce groupe de travail est à la fois distinct et compatible avec le cours de M1-2A avancé « Probabilités de grande dimension » (High dimensional probability).
PSL - Master 2 : A review of probability theory foundations (pre-school)
A pre-school week for second year of Master Mathématiques de l'Assurance de l'Économie et de la Finance (MASEF) and Master Mathématiques Appliquées et THéoriques (MATH). The objective of this pre-school is to recall fundamental aspects of probability theory at the level of a first year of master. This helps to prepare the students for the courses proposed in the master in particular to the course on stochastic calculus. Before the year 2021–2022, this pre-school was essentially the first part of the stochastic calculus course. What was removed (actually postponed to the stochastic calculus course) is the Lebesgue – Stieltjes integral associated to paths of finite variation, the Wiener integral, the Cameron-Martin formula, and its application to exit times, and the Kunita-Watanabe inequality.
- PDF 2021-2022 Rough lecture notes with typos (~65p.)
- Short bibliography:
- Borkar - Probability theory. An advanced course (1995)
- Gut - Probability: a graduate course (2013)
- Revuz and Yor - Continuous martingales and Brownian motion (1999)
PSL - Master 2 : Introduction to Stochastic Calculus
This course belongs to the Master Mathématiques de l'Assurance de l'Économie et de la Finance (MASEF) and Master Mathématiques Appliquées et THéoriques (MATH). It provides an introduction to stochastic calculus: Brownian motion, quadratic variation, Doob stopping and maximal inequalities for martingales, local martingales, stochastic integral, semi-martingales, Itô formula, Lévy characterization of Brownian motion, Girsanov formula, Dubins-Schwarz theorem, stochastic differential equations with Lipschitz coefficients, notion of explosion for locally Lipschitz coefficients, Bessel, Ornstein-Uhlenbeck, and Langevin processes, Markov semigroup and infinitesimal generator, Duhamel formula, strong Markov property, link with Fokker-Planck and heat partial differential evolution equations, Feynman-Kac formula and real Schrödinger operators, probabilistic representation of the Dirichlet problem.
- PDF 2020-2021 Rough lecture notes with typos (~150p.)
- PDF 2020-2021 Exam without solutions
- PDF 2020-2021 Exam with solutions
- PDF 2019-2020 Exam without solutions
- PDF 2019-2020 Exam with solutions
- PDF 2018-2019 Exam without solutions
- PDF 2018-2019 Exam with solutions
- Short bibliography:
- Baudoin - Diffusion Processes and Stochastic Calculus, EMS (2014)
- Evans - An introduction to stochastic differential equations, AMS (2013)
- Le Gall - Brownian Motion, Martingales, and Stochastic Calculus, Springer (2016)
- Ikeda and Watanabe - Stochastic Differential Equations and Diffusion Processes, North-Holland (1981)
- Karatzas and Shreve - Brownian Motion and Stochastic Calculus, Springer (1988)
- Kuo - Introduction to Stochastic Integration, Springer (2005)
- Øksendal - Stochastic Differential Equations: An Introduction with Applications, Springer (2010)
- Archive before 2017 from Halim Doss, vintage, hand written, in French
Dauphine-PSL - Master 2 : Logarithmic Sobolev Inequalities Essentials
This course, in collaboration with Joseph Lehec, belonged to the Master Mathématiques Appliquées et THéoriques (MATH). This course provides a modern overview on logarithmic Sobolev inequalities. These inequalities have been the subject of intense activity in the recent decades in relation with the analysis and geometry of Markov processes and diffusion evolution equations. This course is designed to be accessible to a wide audience. It is divided into seven lectures. The examination will consist in reading a research paper in the field and giving a short talk on it.
This course was also given in Universidad de Chile, Santiago de Chile, in fall 2017, as part of the Programme Régional France - Amérique latine Caraïbe (PREFALC) organized by Stéphane Mischler.
- PDF 2017-2018 Rough lecture notes (~76p.)
- Short bibliography:
- D. Bakry & I. Gentil & M. Ledoux - Analysis and Geometry of Markov Diffusion Operators
- G. Royer - An Initiation to Logarithmic Sobolev inequalities
- C. Ané et al - Sur les inégalités de Sobolev logarithmiques
École Polytechnique - Probabilités (2011-2017)
-
- PDF 2016-2017 Exercices (~90, 20p.)
- PDF 2016-2017 Exercices corrigés (~90)
- PDF 2016-2017 Projet Estimation du paramètre d'une loi exponentielle
- PDF 2016-2017 Projet Estimateur par fenêtres glissantes
- PDF 2015-2016 Projet Marche aléatoire sur le cercle
- PDF 2015-2016 Projet Permutations aléatoires
- PDF 2014-2015 Projet Modèle de Wright-Fisher
- PDF 2014-2015 Projet Agrégation limitée par diffusion interne
- PDF 2013-2014 Projet Marche aléatoire en paysage aléatoire
- PDF 2012-2013 Projet Équation de récurrence linéaire sur un signal
- PDF 2012-2013 Projet Simulation de lois discrètes
- PDF 2012-2013 Projet Modélisation et simulation d'une revue
- PDF 2011-2012 Projet Matrices doublement stochastiques
- PDF 2011-2012 Projet Permutations aléatoires
- PDF 2011-2012 Projet Approximations gaussienne et poissonnienne
- PDF 2011-2012 Projet Processus de taille de fenêtre TCP
-
- PDF 2013-2014 Note à propos des trucs probabilistes élémentaires
- PDF 2013-2014 Note à propos d'une LGN et d'un TCL randomisés
- PDF 2013-2014 Note à propos des chaînes de Markov discrètes
- PDF 2013-2014 Note à propos des temps d'atteinte pour les chaînes de Markov discrètes
- PDF 2011-2012 Exposé Graphes et matrices aléatoires
Dauphine-PSL - Master 1 : Introduction aux séries temporelles
Ce cours faisait partie du Master 1 Mathématiques Appliquées de Dauphine-PSL.
- PDF 2016-2017 Notes de cours avec coquilles (102p.)
- PDF 2016-2017 Exercices de travaux dirigés avec correction (52p.)
- PDF 2016-2017 Annales d'examens avec correction (72p.)
- PDF 2016-2017 Livret (tout en un : notes de cours, exercices et annales corrigés) (228p.)
Marne-la-Vallée & Dauphine-PSL - Master 2 : Stochastic Models
Ce cours faisait partie du Master Mathématiques appliquées - Parcours Analyse et Probabilité. Il a été dispensé (en anglais) pour la dernière fois en 2014-2015. Les notes de cours ci-dessous sont en français.
This course is divided into 7 lectures of 3 hours. Each lecture is devoted to the study of a particular stochastic model. The selected models may vary from one year to another, and the selection for 2013 included Fisher-Wright models, Random walks models, Galton-Watson branching models, Random matrix models, and Growth-fragmentation models. The examination consists in giving a mini-lecture on an article in the spirit of the course. The course is in English, and some lecture notes in French are available below.
- PDF 2013-2014 Notes de cours (109p.) (ancêtre du livre Recueil de modèles stochastiques avec Florent Malrieu)
- PDF 2012-03 Examen mars 2012 Marne (processus de branchement sexué, modèle de Daley)
- PDF 2011-03 Blog Examen mars 2011 Marne (problème de la plus longue sous-suite croissante)
Toulouse & Rennes & Marne-la-Vallée - Cours Master 2 : Modèles markoviens en biologie
Biskra & Oran - Master 1 : Chaînes de Markov
Le cours de Biskra (2006) a été donné en marge d'un colloque sur l'analyse stochastique et ses applications, organisé principalement par Brahim Mezerdi (Université de Biskra) et Boualem Djehiche (KTH Stockholm). Biskra est une ville des Aurès, aux portes du Sahara, dans l'est algérien. Le cours d'Oran (2011) a été donné conjointement avec Amine Asselah, à l'invitation de Setti Ayad (Université d'Oran), pour des étudiants de master d'Oran et de Tlemcen.
- PDF Biskra 2006 Notes de cours (57p. rédigées par DC)
- PDF Oran 2011 Notes de cours de Ferrari et Galves
- PDF Oran 2011 Livre de D. A. Levin-Peres-Wilmer
- PDF Oran 2011 Feuille d'exercices (rédigée par AA)
- PDF Oran 2011 Exemple de convergence abrupte (rédigé par DC)
Marne-la-Vallée - Préparation à l'agrégation interne : probabilités et statistique
Des notes de cours rédigées en collaboration avec Pierre-André Zitt, sous forme de livre, sont disponibles ci-dessus.
Toulouse - Préparation à l'agrégation externe : oral de modélisation option probabilités et statistique (option A)
Ce matériel date de la période 1999-2002, 2003-2005.
- PDF Fragment n°0 - Une initiation à Matlab
- PDF Fragment n°1 - Qu'est-ce que la simulation ?
- PDF Fragment n°2 - Théorèmes limites classiques
- PDF Fragment n°7 - Quelques mots sur l'entropie
- PDF Appendice - Quelques lois classiques
- PDF Feuille de TP n°1 - Initiation Matlab
- PDF Feuille de TP n°2 - Initiation Matlab
- PDF Feuille de TP n°3 - LGN et TLC
- PDF Feuille de TP n°4 - Calcul d'intégrales par méthode de Monte-Carlo
- PDF Feuille de TP n°5 - Espérance conditionnelle en modélisation
- PDF Feuille de TP n°6 - Martingales en modélisation
- PDF Feuille de TP n°7 - Vecteurs aléatoires et modèle linéaire gaussiens
- PDF Feuille de TP n°8 - Tests non paramétriques du chi-deux en modélisation
- PDF Feuille de TP n°9 - Régions de confiance en modélisation
- PDF Feuille de TP n°10 - Fonction de répartition empirique
- PDF Feuille de TP n°11 - Loi exponentielle en modélisation
- PDF Feuille de TP n°12 - Chaînes de Markov à espace au plus dénombrable
- PDF Feuille de TP n°13 - Transformée de Laplace en modélisation
- PDF 2002 Vieux manuel (140p.)
- PDF Texte sur un modèle d'agrégat écrit par Sébastien Blachère (Université de Marseille)
- PDF Texte sur Kaplan-Meier écrit par Jean-François Dupuy (Université de Toulouse III)
Quelques liens
Software (C++, LaTeX, Python, C#, Julia, ...)
- Simcoul Julia code
In collaboration with Grégoire Ferré
Julia code for simulation of Coulomb gases and more with HMC
Git repository on CNRS/PLM GitLab - The w2m.py Python module/script
Python web spider walking through a subset of WWW and producing an adjacency matrix
Module on PyPi Git repository on GitHub - The ejpecp LaTeX2ε class
In collaboration with Krzysztof Burdzy
LaTeX2ε document class for Electronic Journal of Probability and Electronic Communications in Probability
LaTeX Package on CTAN - Computational Rough Paths (CoRoPa)
In collaboration with Terry J. Lyons
C++ template classes libraries handling tree structures and free Lie algebras, including Campbell-Baker-Hausdorff formula, shuffle product, Dynkin map, dynamic Hall bases, etc. Based on STL and compatible with GMP
Webpage and package on SourceForge - The Girko circular law in Python via SageMathCell
Miscellany
You said Miscellany?
- Read and write my name
- On my passports and ID cards, my name is written Djalil Chafaï
- Read Djalil like you read it in Django Reinhardt (thanks Jack!)
- Djalil is my first name and Chafaï is my surname or lastname or family name
- English speaking people should read my name as Jalil Shaf-i-e
- French speaking people should read it as Djè-lil Chaf-aille-i
- Arabic speaking people may read it directly شاﻓﻌﻲ جلیل
- All the rest of the world may read IPA ʃaːfɪʕɪ dʒəlɪl
- For (La)TeX you may write
Djalil Chafa\“{\i} - For ArabTeX you may use
^sAfi`iy ^galiyl - For HTML just write
Djalil Chafa&iuml;
- Numbers
- Favourite prime number is 15061973, the 974461th
- Molecules
- I also taste CH3CH2OH in good wines such as Château Peyrines (F-33410 Cadillac, France)
- Paul Sabatier Reaction: CO2 + 4H2 → CH4 + 2H2O
- Popular science?
- À vrai dire, je n'aime pas beaucoup le terme « vulgarisation » qui a la même racine que le mot péjoratif « vulgaire ». Il faudrait forger un nouveau terme qui signifierait « rendre accessible avec enthousiasme en excitant la curiosité ».
- Social mathematics
- Mathematical collections
- Blogs
- Papers
- Computer Science
- {0,1}∞ on my blog
- Have you heard about the Gödel prize? and the Turing award?
- Probability
- Provocation: « Si tu ne sais pas ce que c'est que les probabilités, c'est probablement ce qui te manque pour comprendre la physique ! »
- « Un homme tirait au sort toutes ses décisions. Il ne lui arriva pas plus de mal qu'aux autres qui réfléchissent. »
Paul Valéry, Choses Tues, Oeuvres II, Pléiade, p. 505.
Denis Talay : « Et s'il avait réfléchi en plus de tirer au sort ? »
- Numerical Analysis
- Volker Strassen, from the law of the iterated logarithm to matrix multiplications
- Mathematics & Biology
- Mathematics & Physics
- «Le physicien traite les problèmes du véhicule à une roue (la brouette), à deux roues (tilbury ou bicyclette), à trois, à quatre roues. Le mathématicien traite le problème général du véhicule à n roues, n étant entier ou fractionnaire, positif ou négatif, réel ou imaginaire.»
Henri Bouasse
- Free software
- « Don't forget that Linux became only possible because 20 years of OS research was carefully studied, analyzed, discussed and thrown away. »
Ingo Molnár - « This is the Unix philosophy: Write programs that do one thing and do it well. Write programs to work together. Write programs to handle text streams, because that is a universal interface. »
Doug McIlroy
- Minds
- Beyond pure science: Jean Bricmont and Noam Chomsky
- Englishman: Harold Pinter
- Middle East: Avraham Burg and Shlomo Sand
- Europe
- Literature (or « lis tes ratures »)
- Michel Houellebecq (1958 - )
- « Alice posait sur nous ce regard à la fois affectueux et légèrement moqueur des femmes qui suivent une conversation entre hommes, cette chose curieuse qui semble toujours hésiter entre la pédérastie et le duel. »
in Soumission p. 58 (2015) - « Il avait un cachet “intellectuel de droite” assez séduisant, me dis-je, ça lui assurait une petite singularité à la fac. On peut laisser parler les gens assez longtemps, ils sont toujours intéressés par leur propre discours, mais il faut quand même relancer de temps en temps, un minimum. »
in Soumission p. 60 (2015)
- Alexandre Soljenitsyne (1918 - 2008)
- « Peu à peu, j’ai découvert que la ligne de partage entre le bien et le mal ne sépare ni les États ni les classes ni les partis, mais qu’elle traverse le cœur de chaque homme et de toute l’humanité. »
- Art
- Pablo Picasso (1881 - 1973)
- « La inspiración existe, pero tiene que encontrarte trabajando » (”Inspiration exists, but it has to find you working)“
in Tomás R. Villasante (1994), Las ciudades hablan: identidades y movimientos sociales en seis metrópolis latinoamericanas. p. 264.
- Guy Ribes (1948 - )
- « Comme pour n'importe quel tableau, chaque faux suppose une recherche. Un peintre, qu'il soit faussaire ou non, est, toute sa vie, un étudiant qui se met à l'école de l'art. Je n'ai pas fait un seul Matisse ou un seul Renoir, je les ai faits en dix exemplaires, jusqu'à ce que je comprenne leur nature et leur sens. On ne peut pas créer une œuvre de cette importance du premier coup. On commet des erreurs, on détruit, on recommence… »
in Autoportrait d'un faussaire p. 120 (2015)
- Mathematicians and physicists
- Free probability: Eugene P. Wigner and John von Neumann were schoolmates in Budapest and colleagues in Princeton. Their works were connected by Dan-Virgil Voiculescu, a romanian-american mathematician.
- Didier Concordet (1962 - )
- «D'année en année, les étudiants sont de plus en plus jeunes. »
- Roger-Dominique Bakry (1954 - × )
- « Y'a qu'un nombre fini d'idées. »
- « À part Cauchy-Schwarz… » and more recently « À part Pythagore… »
- « Ce qui compte, en maths, c'est les idées »
- « En général, dans un papier, entre l'abstract et le non-sense, il y a l'introduction »
- « De toute manière, tous les papiers sont faux, et ça n'est pas grave, car personne ne les lit »
- « Tu ferais mieux de te trouver un vrai métier… »
- James Clerk Maxwell (1831 - 1879)
- «… They say that Understanding ought to work by the rules of right reason. These rules are, or ought to be, contained in Logic; but the actual science of Logic is conversant at present only with things either certain, impossible, or entirely doubtful, none of which (fortunately) we have to reason on. Therefore the true Logic for this world is the Calculus of Probabilities, which takes account of the magnitude of the probability (which is, or which ought to be in a reasonable man's mind). This branch of Math., which is generally thought to favour gambling, dicing, and wagering, and therefore highly immoral, is the only “Mathematics for Practical Men,” as we ought to be. … James Clerk Maxwell (1850) Letter to Lewis Campbell; reproduced in L. Campbell and W. Garrett, The Life of James Clerk Maxwell, Macmillan, 1881, page 80.
- Mark Kac (1914 - 1984)
- «I prefer concrete things and I don't like to learn more about abstract stuff than I absolutely have to. »
- Henri Poincaré (1854 - 1912)
- À propos de la loi normale du théorème central limite, désigné sous le nom de loi des erreurs à l'époque : « Cette loi ne s'obtient pas par des déductions rigoureuses ; plus d’une démonstration qu’on a voulu en donner est grossière, entre autres celle qui s’appuie sur l’affirmation que la probabilité des écarts est proportionnelle aux écarts. Tout le monde y croit cependant, me disait un jour M. Lippmann, car les expérimentateurs s’imaginent que c’est un théorème de mathématiques, et les mathématiciens que c’est un fait expérimental. » In Le calcul des Probabilités (1896) p. 149). Comme le dit le physicien Oriol Bohigas, de nos jours, nous savons que c'est à la fois un fait expérimental et un théorème de mathématiques !
- Bruno de Finetti (1906 - 1985)
- «…Mathematics is not by now a field already explored, just to learn and pass on to posterity as it is. It is always progressing, it is enriching and lightening itself, it is a lively and vital creature, in full development and just for these reasons I love it, I study it and I wish to devote my life to it…»
- Philippe Biane (1962 - )
- Les probabilités et le mouvement brownien (Vidéo, magnifique cravate)
- Vladimir Arnold (1937 - 2010)
- La mathématique expérimentale (Vidéo)
- « Les mathématiques ne sont qu'un chapitre de la physique ! »
- Jean-Pierre Serre (1926 - )
- How to write mathematics badly (Video)
- Bernard Beauzamy (1947 - )
- Les erreurs en mathématiques (Vidéo)
-
- Jean-Philippe Bouchaud (1962 - )
-
- Bernard Derrida (1952 - )
- Physique statistique (Vidéo)
-
- « There are several categories of scientists in the world; those of second or third rank do their best but never get very far. Then there is the first rank, those who make important discoveries, fundamental to scientific progress. But then there are the geniuses, like Galilei and Newton. Majorana was one of these. » 1938, about Ettore Majorana
- Ludwig Boltzmann (1844 - 1906)
- « … If you ask me about my innermost conviction whether our century will be called the century of iron or the century of steam or electricity, I answer without hesitation: It will be called the century of the mechanical view of Nature, the century of Darwin. … »
- John Forbes Nash Jr. (1928 - 2015)
- « The ideas I had about supernatural beings came to me the same way that my mathematical ideas did. So I took them seriously. »
- Gian-Carlo Rota (1932 - 1999)
- Ten Lessons I Wish I Had Been Taught Notices on the AMS 44 (1997)
- « Don't fight with success »
- « You should only listen to advice that you do not have to follow »
- Paul Halmos (1916 - 2006)
- « Don't just read it; fight it! Ask your own questions, look for your own examples, discover your own proofs. Is the hypothesis necessary? Is the converse true? What happens in the classical special case? What about the degenerate cases? Where does the proof use the hypothesis? »
- Godfrey Harold Hardy (1877 - 1947)
- « The mathematician’s patterns, like the painter’s or the poet’s must be beautiful; the ideas like the colours or the words, must fit together in a harmonious way. Beauty is the first test: there is no permanent place in the world for ugly mathematics. »
- John von Neumann (1903 - 1957)
- «If people do not believe that mathematics is simple, it is only because they do not realize how complicated life is. »
-
- « Young men should prove theorems, old men should write books. »
- Hermann Weyl (1885 - 1955)
- « My work always tried to unite the truth with the beautiful, but when I had to choose one or the other, I usually chose the beautiful. »
- Edward Brian Davies (1944 -)
- « Studying non-self-adjoint operators is like being a vet rather than a doctor: one has to acquire a much wider range of knowledge, and to accept that one cannot expect to have as high a rate of success when confronted with particular cases. It comprises a collection of methods, each of which is useful for some class of such operators. »
- Daniel W. Stroock (1940 -)
- « Mathematics is one, and possibly the only, human endeavor for which there is a widely, if not universally, recognized criterion with which to determine truth. For this reason, mathematicians can avoid some of the interminable disputes which plague other fields. On the other hand, I sometimes wonder whether the most interesting questions are not those for which such disputes are inevitable. »
- Terence Chi-Shen Tao (陶哲軒) (1975 - )
- Sir William Timothy Gowers (1963 - )
- William P. Thurston (1946 - )
- Others
- Georges Brassens (1921 - 1981)
- « Mais sans technique, un don n'est rien ♫ Qu'une sale manie… » in Le mauvais sujet repenti
- Antonio Gramsci (1891 - 1937) (also attributed to Romain Rolland)
- « I’m a pessimist because of intelligence, but an optimist because of will. » in Letter from Prison (19 December 1929);
- Audrey Dussutour (1977 - )
- « La recherche est comme une plante, dont la fleur serait la recherche appliquée, et la racine la recherche fondamentale. »
- Some curiosities
- How to select the number of classes in a histogram? Californian way and Parisian way
- Money
- Who said that mathematicians do not like money? It seems at least that the converse is false…
